Physics presentation: interactions

Yes, beer is good. (Perhaps there's an extra letter in this sign that shouldn't be there...) But if you walked into a microbrewery with a wide selection of fine beers on tap, how do you know which beer is good? You don't have the time, money, or wherewithal to order and drink a pint of each different type of beer. So what do you do?

Some microbreweries offer what they call a "Brew-Ski," where a small sample of each of their beers on tap is placed, literally, on a "ski," in order to introduce you to their line-up. (These samplers are sometimes called "flights.")

Sampling individual beers separately is fine, but what would happen if all the different beers from a "Brew-Ski" were combined into the same glass? Is this so wrong?

Which leads us to our discussion of different types of mechanical interactions--forces. Since we will investigate the details of these forces later in this (algebra-based college physics) course to, let's settle for the "Brew-Ski" approach, where we'll look at a selection of important forces, briefly. And since many situations in the real world involve more than one type of force acting on the same object at the same time, we'll consider the result of combining different types of beers--that is, forces--into a net force.

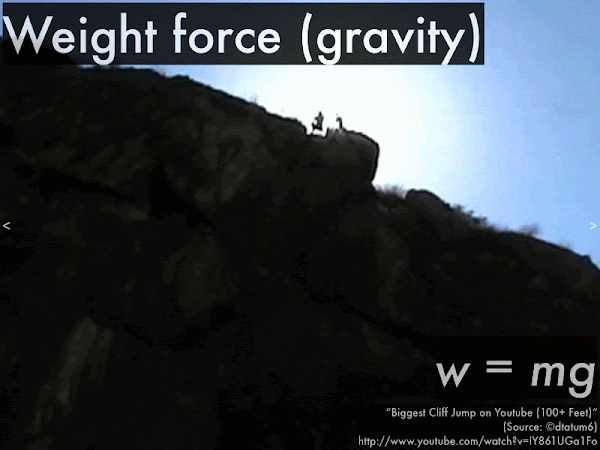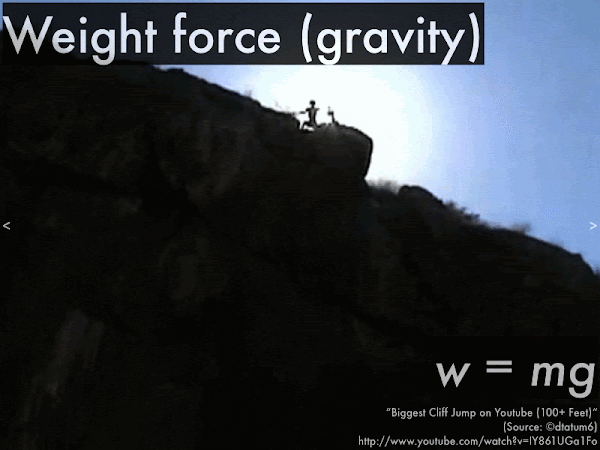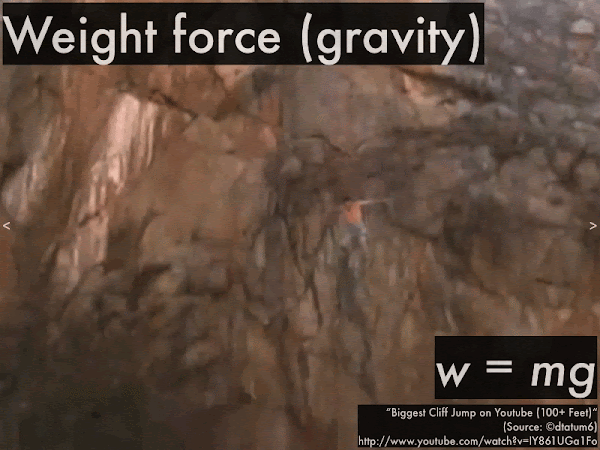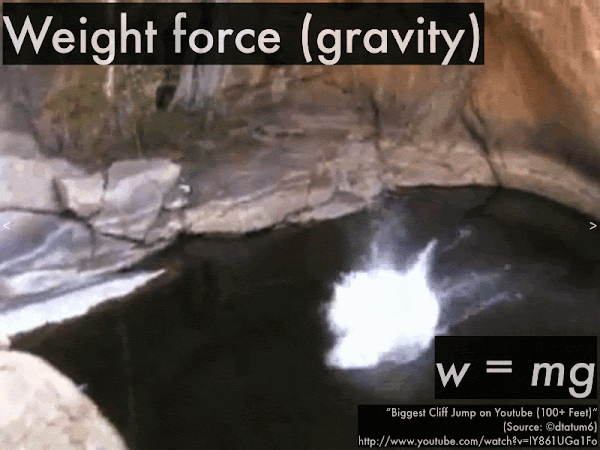
Here's our "Brew-Ski" force line-up. Remember, we'll go into more detail on each of these as the course progresses, but for now, this will just be an introductory tasting.

Weight, or the force of gravity, is the easy-cheesy force. For our purposes its magnitude is always equal to the product of the object's mass m, and the gravitational strength constant g ("acceleration due to gravity"), regardless of the motion or location of the object. (Movie link: "Biggest Cliff Jump on Youtube (100+ Feet).")

The normal force is the supporting contact force exerted by a surface. Its magnitude can vary from zero (no or barely any contact) up to ∞, but practically speaking a surface can only exert up to a maximum value, depending on structural integrity of the underlying material, until it fails. In this case, the floor can exert a normal force to support the weight of the contents of the room, but only up to when the room gets filled with too much water. (Movie link: "Collapsing floor by filling room with water.")

The tension force is the force exerted along a string, rope, or cable. Its magnitude can vary from zero (slack or barely pulling) up to ∞, but practically speaking a rope will have a maximum value, depending on the strength of its material/construction, until it fails. Here, a towing "snatch" strap exerts a tension force to pull on a stuck vehicle, but only up to when it is pulled too much. (Movie link: "Broken snatch strap.")

The static and kinetic friction forces apply to unsticking or subsequently sliding an object across a surface, respectively. The magnitude of the static friction force can vary from zero (no or barely trying to unstick an object) up to a maximum value, at which point the object becomes unstuck, and just begins to move. The magnitude of the kinetic friction force magnitude is always a constant value, once the object is already unstuck and moving. Note that the maximum magnitude of "stiction" (static friction) is greater than the magnitude of "sliption" (kinetic friction). (Movie link: "Static vs. Sliding Friction.")

So much for the "Brew-Ski" overview of different types of forces. Let's move on to more complex situations, and the tools used to handle them.

Most everyday situations involve more than one type of interaction happening at the same time--consider the forces acting on the board: weight, normal force, tension, static friction force (assuming that the girl is not sliding off of the board), and kinetic friction force (assuming that the board is already sliding across the sand).

Each force is represented with a vector--the magnitude (length) represents the strength of the interaction, and the direction represents, well, the direction of the force exerted. Typically many forces, all acting on the same object can be depicted on a free body diagram.

Forces all acting on the same object all add together to result in a net force (F_net), which is what the summation operator signifies. Since mathematically vectors can be broken up into x- and y- components, and then separately added together to find the resulting x- and y- components of the net force.

Looking ahead, how many Newton's laws are there? (Three.) But there are only two ways to classify motion--constant, or changing, corresponding to Newtons first law, or second law. Or only two ways to classify net force--zero, or non-zero, corresponding to Newton's first law, or second law.

So if there's only two types of motion, and two types of net forces, what's up with Newton's third law? As it turns out, Newton's third law has nothing to do with motion or net force, but something else entirely, something much more universal and encompassing than considering a particular type of motion or net force...