Astronomy 10 Quiz 10, Fall Semester 2007
Cuesta College, San Luis Obispo, CA
Astronomy 10 learning goal Q10.3
[3.0 points.] Which one of the following choices best describes a close pair (mass-exchanging) binary system that can have repeatable x-ray bursts?
(A) Two neutron stars.
(B) A massive main sequence star and a red dwarf.
(C) A black hole and a neutron star.
(D) A supergiant and a neutron star.
(E) A supergiant and a black hole.
Correct answer: (D)
The hydrogen shed from a supergiant will form a degenerate layer around a neutron star companion, and this outer layer will undergo fusion, producing an x-ray burst.
Student responses
Section 0135
(A) : 5 students
(B) : 3 students
(C) : 10 students
(D) : 9 students
(E) : 5 students
Student responses
Section 1073
(A) : 7 students
(B) : 3 student
(C) : 6 students
(D) : 22 students
(E) : 5 students
Previous post: Astronomy quiz question: novae and type Ia supernovae
20071129
Astronomy midterm question: giant end-stage
Astronomy 10 Midterm 3, Fall Semester 2007
Cuesta College, San Luis Obispo, CA
Astronomy 10 learning goal M3.1
[15 points.] Why can't a medium mass star live as a giant forever after its main sequence lifetime ends? Discuss why all giants must eventually become planetary nebulae and white dwarfs, by explaining the properties of giants and stellar evolution.
Solution and grading rubric:
Section 1073
p: 10 students
r: 8 students
t: 17 students
v: 4 students
x: 3 students
y: 0 students
z: 0 students
A sample of a "p" response (from student 0115) is shown below:
Another "p" response (from student 4040):
Cuesta College, San Luis Obispo, CA
Astronomy 10 learning goal M3.1
[15 points.] Why can't a medium mass star live as a giant forever after its main sequence lifetime ends? Discuss why all giants must eventually become planetary nebulae and white dwarfs, by explaining the properties of giants and stellar evolution.
Solution and grading rubric:
- p = 15/15:
Correct. In its giant phase, heavier and heavier elements are fused until the core becomes carbon, but temperatures and pressures are unable to continue heavier fusion. Without an energy source to balance gravity, the giant lifetime of the medium mass star ends as the core gravitationally contracts into a white dwarf, while giving off enough energy to disperse its outer layers as a planetary nebula. - r = 12/15:
Nearly correct (explanation weak, unclear or only nearly complete); includes extraneous/tangential information; or has minor errors. Understands how the giant lifetime of a medium mass star occurs, but does not completely explain why it must end. - t = 9/15:
Contains right ideas, but discussion is unclear/incomplete or contains major errors. May confuse discussion with that of supergiants and type II supernovae, but at least understands the importance of no more energy being released from fusion. - v = 6/15:
Limited relevant discussion of supporting evidence of at least some merit, but in an inconsistent or unclear manner. - x = 3/15:
Implementation/application of ideas, but credit given for effort rather than merit. - y = 1.5/15:
Irrelevant discussion/effectively blank. - z = 0/15:
Blank.
Section 1073
p: 10 students
r: 8 students
t: 17 students
v: 4 students
x: 3 students
y: 0 students
z: 0 students
A sample of a "p" response (from student 0115) is shown below:
Another "p" response (from student 4040):
20071128
Astronomy midterm question: white dwarf and supergiant cluster
Astronomy 10 Midterm 3, Fall Semester 2007
Cuesta College, San Luis Obispo, CA
Astronomy 10 learning goal Q9.5
[15 points.] Why is it not possible to have both white dwarfs and supergiants in the same star cluster? Explain using the properties of mass and stellar lifetimes.
Solution and grading rubric:
Section 1073
p: 8 students
r: 10 students
t: 7 students
v: 6 students
x: 10 students
y: 1 student
z: 0 students
A sample of a "p" response (from student 4040) is shown below:
Cuesta College, San Luis Obispo, CA
Astronomy 10 learning goal Q9.5
[15 points.] Why is it not possible to have both white dwarfs and supergiants in the same star cluster? Explain using the properties of mass and stellar lifetimes.
Solution and grading rubric:
- p = 15/15:
Correct. White dwarfs are the end product of medium mass stars. Supergiants are near the end of massive stars. It will take longer for a medium mass protostar to reach the main sequence, end its main sequence lifetime, go through its giant phase to become a white dwarf than a massive protostar to go through similar stages. Thus stars in a star cluster, which started at the same time, cannot have white dwarfs and supergiants existing at a same later time. - r = 12/15:
Nearly correct (explanation weak, unclear or only nearly complete); includes extraneous/tangential information; or has minor errors. Understands that evolution times (protostar to main sequence, main sequence lifetime, main sequence to end stage) are inversely proportional to mass, but discussion of these stages has minor omissions or errors. Or understands evolutionary sequence that results in these end stages, but discussion of evolutionary times has minor omissions or errors. - t = 9/15:
Contains right ideas, but discussion is unclear/incomplete or contains major errors. As (r), but discussion of evolutionary stages, or times is problematic. - v = 6/15:
Limited relevant discussion of supporting evidence of at least some merit, but in an inconsistent or unclear manner. - x = 3/15:
Implementation/application of ideas, but credit given for effort rather than merit. - y = 1.5/15:
Irrelevant discussion/effectively blank. - z = 0/15:
Blank.
Section 1073
p: 8 students
r: 10 students
t: 7 students
v: 6 students
x: 10 students
y: 1 student
z: 0 students
A sample of a "p" response (from student 4040) is shown below:
20071127
Astronomy midterm question: star size comparison
Astronomy 10 Midterm 3, Fall Semester 2007
Cuesta College, San Luis Obispo, CA
Astronomy 10 learning goal Q8.3
[15 points.] Consider the blackbody (continuous) spectra of two different objects, on the same intensity versus wavelength graph shown below. Explain using Wien's law and/or the Stefan-Boltzmann law why object X is smaller than object Y.
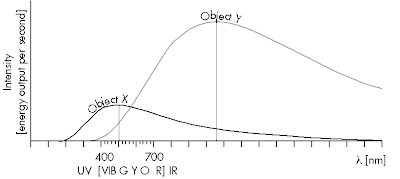Solution and grading rubric:
Section 1073
p: 14 students
r: 4 students
t: 14 students
v: 9 students
x: 0 students
y: 0 students
z: 1 student
A sample of a "p" response (from student 4888) is shown below:
Another "p" response appeals to analyzing the Stefan-Boltzmann equation (from student 7276):
A few students (7635 shown here), upon immediately receiving the midterm at the start of class, had written down from short-term memory on the cover page the six special comparison cases discussed in class using the Stefan-Boltzmann law:
Cuesta College, San Luis Obispo, CA
Astronomy 10 learning goal Q8.3
[15 points.] Consider the blackbody (continuous) spectra of two different objects, on the same intensity versus wavelength graph shown below. Explain using Wien's law and/or the Stefan-Boltzmann law why object X is smaller than object Y.
Solution and grading rubric:- p = 15/15:
Correct. Wien's law says that Object X (peak intensity at a shorter wavelength) must be hotter than Object Y. The Stefan-Boltzmann law says that luminosity (related to the intensity of the peak) is proportional to size and temperature^4. Since Object X is hotter, in order for it to be less luminous than Object Y is for Object X to be much smaller than Object Y. May only implicitly utilize Wien's law in discussion. - r = 12/15:
Nearly correct (explanation weak, unclear or only nearly complete); includes extraneous/tangential information; or has minor errors. - t = 9/15:
Contains right ideas, but discussion is unclear/incomplete or contains major errors. At least understands the Stefan-Boltzmann law. - v = 6/15:
Limited relevant discussion of supporting evidence of at least some merit, but in an inconsistent or unclear manner. - x = 3/15:
Implementation/application of ideas, but credit given for effort rather than merit. - y = 1.5/15:
Irrelevant discussion/effectively blank. - z = 0/15:
Blank.
Section 1073
p: 14 students
r: 4 students
t: 14 students
v: 9 students
x: 0 students
y: 0 students
z: 1 student
A sample of a "p" response (from student 4888) is shown below:
Another "p" response appeals to analyzing the Stefan-Boltzmann equation (from student 7276):
A few students (7635 shown here), upon immediately receiving the midterm at the start of class, had written down from short-term memory on the cover page the six special comparison cases discussed in class using the Stefan-Boltzmann law:
20071126
Astronomy midterm question: star cluster age
Astronomy 10 Midterm 3, Fall Semester 2007Cuesta College, San Luis Obispo, CA
Astronomy 10 learning goal Q9.5
[15 points.] Shown at right is an H-R diagram of a star cluster, where all of these stars have the same age. Discuss whether this star cluster is very young or is very old, and explain why.
Solution and grading rubric:
- p = 15/15:
Correct. Understands that low mass stars evolve slower than massive stars, and that since they have reached the main sequence line, after the massive stars have come and left, this must be a very old star cluster. May use the turnoff point on main sequence line. - r = 12/15:
Nearly correct (explanation weak, unclear or only nearly complete); includes extraneous/tangential information; or has minor errors. Understands that the two stars must have the same temperature, but typically misapplies the Stefan-Boltzmann law. - t = 9/15:
Contains right ideas, but discussion is unclear/incomplete or contains major errors. At least understands evolutionary stages of stars, but determines cluster to be very young. - v = 6/15:
Limited relevant discussion of supporting evidence of at least some merit, but in an inconsistent or unclear manner. Argument based on temperature or luminosity of stars. - x = 3/15:
Implementation/application of ideas, but credit given for effort rather than merit. - y = 1.5/15:
Irrelevant discussion/effectively blank. - z = 0/15:
Blank.
Section 0135
p: 19 students
r: 4 students
t: 6 students
v: 5 students
x: 1 student
y: 0 students
z: 0 students
A sample of a "p" response (from student 1338) is shown below:
Another "p" response (from student 4136):
20071123
Astronomy midterm question: comparing same-color stars
Astronomy 10 Midterm 3, Fall Semester 2007
Cuesta College, San Luis Obispo, CA
Astronomy 10 learning goal Q8.5
[15 points.] Consider the following statement: "If two stars have the same color, the brighter star will be bigger." Discuss whether this statement is true or not, and support your answer using Wien's law and/or the Stefan-Boltzmann law.
Solution and grading rubric:
Section 0135
p: 15 students
r: 3 students
t: 8 students
v: 9 students
x: 0 students
y: 0 students
z: 0 students
A sample of a "p" response (from student 9389) is shown below:
Another sample of a "p" response (from student 3158):
A "p" response that uses a comparative sample of four stars, as was done in class (from student 7649):
Cuesta College, San Luis Obispo, CA
Astronomy 10 learning goal Q8.5
[15 points.] Consider the following statement: "If two stars have the same color, the brighter star will be bigger." Discuss whether this statement is true or not, and support your answer using Wien's law and/or the Stefan-Boltzmann law.
Solution and grading rubric:
- p = 15/15:
Correct. Wien's law says that same color (i.e., same peak wavelength) means same temperature. The Stefan-Boltzmann law says that for the same temperature, a brighter luminosity corresponds to a larger size. Thus the statement is true. (May only implicitly utilize one of the two laws in discussion.) - r = 12/15:
Nearly correct (explanation weak, unclear or only nearly complete); includes extraneous/tangential information; or has minor errors. Understands that the two stars must have the same temperature, but typically misapplies the Stefan-Boltzmann law. - t = 9/15:
Contains right ideas, but discussion is unclear/incomplete or contains major errors. At least attempts some systematic application of Wien's law and/or the Stefan-Boltzmann law. - v = 6/15:
Limited relevant discussion of supporting evidence of at least some merit, but in an inconsistent or unclear manner. May involve discussion of apparent versus absolute magnitude. - x = 3/15:
Implementation/application of ideas, but credit given for effort rather than merit. - y = 1.5/15:
Irrelevant discussion/effectively blank. - z = 0/15:
Blank.
Section 0135
p: 15 students
r: 3 students
t: 8 students
v: 9 students
x: 0 students
y: 0 students
z: 0 students
A sample of a "p" response (from student 9389) is shown below:
Another sample of a "p" response (from student 3158):
A "p" response that uses a comparative sample of four stars, as was done in class (from student 7649):
20071121
Physics quiz question: standing wave string tension
Physics 5A Quiz 6, Fall Semester 2007
Cuesta College, San Luis Obispo, CA
Cf. Giambattista/Richardson/Richardson, Physics, 1/e, Problem 11.48
[3.0 points.] Consider a guitar's A-string of a length 0.80 m and is stretched to a tension of 40 N. It vibrates at a fundamental frequency of 220 Hz. If the tension in the string is increased by a factor of 2.0, what happens to the fundamental frequency of this string?
(A) It increases by a factor of 1.4.
(B) It increases by a factor of 2.0.
(C) It increases by a factor of 4.0.
(D) (The fundamental frequency remains the same.)
Correct answer: (A)
The fundamental frequency of a standing wave on a string is:
f_1 = v/(2*L),
where the speed of waves along the string depends on the tension F and linear mass density mu:
v = sqrt(F/mu).
Increasing the tension F by a factor of 2.0 increases the wave speed by a factor of sqrt(2.0) = 1.4, which increases the fundamental frequency of the string by that same 1.4 factor.
Student responses
Sections 0906, 0907
(A) : 7 students
(B) : 7 students
(C) : 3 students
(D) : 4 students
Cuesta College, San Luis Obispo, CA
Cf. Giambattista/Richardson/Richardson, Physics, 1/e, Problem 11.48
[3.0 points.] Consider a guitar's A-string of a length 0.80 m and is stretched to a tension of 40 N. It vibrates at a fundamental frequency of 220 Hz. If the tension in the string is increased by a factor of 2.0, what happens to the fundamental frequency of this string?
(A) It increases by a factor of 1.4.
(B) It increases by a factor of 2.0.
(C) It increases by a factor of 4.0.
(D) (The fundamental frequency remains the same.)
Correct answer: (A)
The fundamental frequency of a standing wave on a string is:
f_1 = v/(2*L),
where the speed of waves along the string depends on the tension F and linear mass density mu:
v = sqrt(F/mu).
Increasing the tension F by a factor of 2.0 increases the wave speed by a factor of sqrt(2.0) = 1.4, which increases the fundamental frequency of the string by that same 1.4 factor.
Student responses
Sections 0906, 0907
(A) : 7 students
(B) : 7 students
(C) : 3 students
(D) : 4 students
20071120
Physics quiz question: spring constant of mass-spring system
Physics 5A Quiz 6, fall semester 2007
Cuesta College, San Luis Obispo, CA
Cf. Giambattista/Richardson/Richardson, Physics, 1/e, Problem 10.32(a)
A 0.20 kg object attached to a horizontal spring oscillates left to right on a frictionless surface with a frequency of 3.5 Hz. The spring constant is:
(A) 16 N/m.
(B) 22 N/m.
(C) 97 N/m.
(D) 4.8×102 N/m.
Correct answer (highlight to unhide): (C)
The period and frequency of a mass-spring system are inverses of each other:
T = (2·π)·√(m/k),
f = 1/T = √(k/m)/(2·π).
Then the spring constant will be given by:
k = m·(2·π·f)2,
k = (0.20 kg)·(2·π·(3.5 Hz))2 = 96.72212313 N/m,
which to two significant figures is 97 N/m.
(Response (A) is (1/m)(2·π/f)2; response (B) is 2·π·f, which is the angular frequency ω; while response (D) is (2·π·f)2, the angular frequency squared.)
Student responses
Sections 0906, 0907
Exam code: quiz06w4v3
(A) : 3 students
(B) : 5 students
(C) : 27 students
(D) : 2 students
Success level: 72%
Discrimination index (Aubrecht & Aubrecht, 1983): 0.55
Cuesta College, San Luis Obispo, CA
Cf. Giambattista/Richardson/Richardson, Physics, 1/e, Problem 10.32(a)
A 0.20 kg object attached to a horizontal spring oscillates left to right on a frictionless surface with a frequency of 3.5 Hz. The spring constant is:
(A) 16 N/m.
(B) 22 N/m.
(C) 97 N/m.
(D) 4.8×102 N/m.
Correct answer (highlight to unhide): (C)
The period and frequency of a mass-spring system are inverses of each other:
T = (2·π)·√(m/k),
f = 1/T = √(k/m)/(2·π).
Then the spring constant will be given by:
k = m·(2·π·f)2,
k = (0.20 kg)·(2·π·(3.5 Hz))2 = 96.72212313 N/m,
which to two significant figures is 97 N/m.
(Response (A) is (1/m)(2·π/f)2; response (B) is 2·π·f, which is the angular frequency ω; while response (D) is (2·π·f)2, the angular frequency squared.)
Student responses
Sections 0906, 0907
Exam code: quiz06w4v3
(A) : 3 students
(B) : 5 students
(C) : 27 students
(D) : 2 students
Success level: 72%
Discrimination index (Aubrecht & Aubrecht, 1983): 0.55
20071119
Astronomy in-class activity: OBAFGKM poetry slam
Astronomy 10, Fall Semester 2007
Cuesta College, San Luis Obispo, CA
Astronomy 10 learning goal Q8.5
Students were instructed to use at least OBAFGKM, and/or part or all of the additional OBAFGKMRNSC or OBAFGKMLT extensions to individually write an original, coherent and an appropriate (nothing worse than "PG-13" rated!) mnemonic, and to give a rousing reading of their OBAFGKM mnemonic poem for the class.
Some favorites from this semester:
Order Beer And Forget your Grades and Keeping (your) Money.
--C. B.
Open Bag And Feel Gold, King Midas
--C. B. (2)
One Boy Accidentally Found Grandpa's Kinky Magazine
--K. D.
Oleanders bloom.
Awkward feelings grow.
Kiss me.
--J. H.
Over-Bearing And Fiesty Girls Kiss More.
--J. H. (2)
Obama Believes Against Foreign Gas and Killing Muslims
--M. N.
Old But Agile Female Gladiators Kill Mockers
--S. O.
Old Bachelors Are Fat Gross Kissing Machines
--D. R.
Orbiting Big Asteroids, Freakishly Ginormous Krypton Masses Radiate Nauseous Substances
--M. W.
Overstuffed Bras Are Fabulous Guy-Knocking Machines, Timber!
--H. L.
Previous post: OBAFGKM poetry slam (Summer Session 2007).
Cuesta College, San Luis Obispo, CA
Astronomy 10 learning goal Q8.5
Students were instructed to use at least OBAFGKM, and/or part or all of the additional OBAFGKMRNSC or OBAFGKMLT extensions to individually write an original, coherent and an appropriate (nothing worse than "PG-13" rated!) mnemonic, and to give a rousing reading of their OBAFGKM mnemonic poem for the class.
Some favorites from this semester:
Order Beer And Forget your Grades and Keeping (your) Money.
--C. B.
Open Bag And Feel Gold, King Midas
--C. B. (2)
One Boy Accidentally Found Grandpa's Kinky Magazine
--K. D.
Oleanders bloom.
Awkward feelings grow.
Kiss me.
--J. H.
Over-Bearing And Fiesty Girls Kiss More.
--J. H. (2)
Obama Believes Against Foreign Gas and Killing Muslims
--M. N.
Old But Agile Female Gladiators Kill Mockers
--S. O.
Old Bachelors Are Fat Gross Kissing Machines
--D. R.
Orbiting Big Asteroids, Freakishly Ginormous Krypton Masses Radiate Nauseous Substances
--M. W.
Overstuffed Bras Are Fabulous Guy-Knocking Machines, Timber!--H. L.
Previous post: OBAFGKM poetry slam (Summer Session 2007).
20071116
Physics clicker question: pendulum period miscalculation
Physics 5A, Fall Semester 2007
Cuesta College, San Luis Obispo, CA
Cf. Giambattista/Richardson/Richardson, Physics, 1/e, Problem 10.60
Students were asked the following clicker question (Classroom Performance System, einstruction.com) near the end of their learning cycle:
[0.6 participation points.] A mass of weight 10.0 N has a period of 1.0 s. The length of the pendulum string is _____ m.
Sections 0906, 0907
"0.25" : 20 students
"24" : 7 students
"10" : 3 students
"5": 2 students
(Other responses, 1 student each: "0.06," "1.02," "2," "3.2," "21.7")
Correct answer: 0.25 m
The period of a pendulum is given by:
T = 2*pi*sqrt(L/g).
Note that the weight, or mass of the pendulum bob does not matter. Solving for L:
L = g*(T/(2*pi))^2.
However, many students did not watch how the order of operations were performed on their graphing calculators. Typically they entered the following:
1/2*pi
ANS^2
ANS*9.8
Because of the factor of pi multiplying the 0.5 evaluated just before it, this results in the incorrect answer of 24 (m?!?), which dangerously resembles the correct answer, apparently only with a misplaced decimal error ("did we need to convert to centimeters?").
In order to force the pi to be evaluated in the denominator, parentheses could be used (or each step could be executed separately, step-by-step):
1/(2*pi)
ANS^2
ANS*9.8
This gives the correct answer of 0.25 m.
Cuesta College, San Luis Obispo, CA
Cf. Giambattista/Richardson/Richardson, Physics, 1/e, Problem 10.60
Students were asked the following clicker question (Classroom Performance System, einstruction.com) near the end of their learning cycle:
[0.6 participation points.] A mass of weight 10.0 N has a period of 1.0 s. The length of the pendulum string is _____ m.
Sections 0906, 0907
"0.25" : 20 students
"24" : 7 students
"10" : 3 students
"5": 2 students
(Other responses, 1 student each: "0.06," "1.02," "2," "3.2," "21.7")
Correct answer: 0.25 m
The period of a pendulum is given by:
T = 2*pi*sqrt(L/g).
Note that the weight, or mass of the pendulum bob does not matter. Solving for L:
L = g*(T/(2*pi))^2.
However, many students did not watch how the order of operations were performed on their graphing calculators. Typically they entered the following:
1/2*pi
ANS^2
ANS*9.8
Because of the factor of pi multiplying the 0.5 evaluated just before it, this results in the incorrect answer of 24 (m?!?), which dangerously resembles the correct answer, apparently only with a misplaced decimal error ("did we need to convert to centimeters?").
In order to force the pi to be evaluated in the denominator, parentheses could be used (or each step could be executed separately, step-by-step):
1/(2*pi)
ANS^2
ANS*9.8
This gives the correct answer of 0.25 m.
20071115
Physics quiz question: buoyant force
Physics 5A Quiz 5, Fall Semester 2007
Cuesta College, San Luis Obispo, CA
Cf. Giambattista/Richardson/Richardson, Physics, 1/e, Problem 9.37, Comprehensive Problem 9.74
[3.0 points.] An aluminum cylinder (of density 2.70 x 10^3 kg/m^3) weighs 8.0 N, and has a volume of 3.0 x 10^(-4) m^3. If the cylinder is suspended from a scale while completely submerged in a fluid, the scale reading is 5.5 N. What is the density of the fluid?
(A) 8.5 x 10^2 kg/m^3.
(B) 1.9 x 10^3 kg/m^3.
(C) 2.7 x 10^3 kg/m^3.
(D) 4.6 x 10^3 kg/m^3.
Correct answer: (A)
From Newton's first law, the upwards bouyant force and tension force must be equal in magnitude to the downwards weight force:
F_B + T = w.
From the definition of the bouyant force, since the object is completely submerged, F_B = rho_fluid*g*Vol, thus:
rho_fluid = (w - T)/(g*Vol).
Choice (B) is T/(g*Vol); choice (B) is w/(g*Vol), which is the density of aluminum; while choice (D) is (w + T)/(g*Vol), which would make the fluid denser than aluminum.
Student responses
Sections 0906, 0907
(A) : 8 students
(B) : 17 students
(C) : 7 students
(D) : 7 students
Cuesta College, San Luis Obispo, CA
Cf. Giambattista/Richardson/Richardson, Physics, 1/e, Problem 9.37, Comprehensive Problem 9.74
[3.0 points.] An aluminum cylinder (of density 2.70 x 10^3 kg/m^3) weighs 8.0 N, and has a volume of 3.0 x 10^(-4) m^3. If the cylinder is suspended from a scale while completely submerged in a fluid, the scale reading is 5.5 N. What is the density of the fluid?
(A) 8.5 x 10^2 kg/m^3.
(B) 1.9 x 10^3 kg/m^3.
(C) 2.7 x 10^3 kg/m^3.
(D) 4.6 x 10^3 kg/m^3.
Correct answer: (A)
From Newton's first law, the upwards bouyant force and tension force must be equal in magnitude to the downwards weight force:
F_B + T = w.
From the definition of the bouyant force, since the object is completely submerged, F_B = rho_fluid*g*Vol, thus:
rho_fluid = (w - T)/(g*Vol).
Choice (B) is T/(g*Vol); choice (B) is w/(g*Vol), which is the density of aluminum; while choice (D) is (w + T)/(g*Vol), which would make the fluid denser than aluminum.
Student responses
Sections 0906, 0907
(A) : 8 students
(B) : 17 students
(C) : 7 students
(D) : 7 students
20071114
Physics quiz question: champagne cork in bottleneck
Physics 5A Quiz 5, Fall Semester 2007
Cuesta College, San Luis Obispo, CA
Cf. Giambattista/Richardson/Richardson, Physics, 1/e, Problem 9.78
[3.0 points.] The pressure inside a bottle of champagne is 5.0 atm higher than the air pressure outside. The neck of the bottle has an inner radius of 1.2 cm. What is the frictional force on the cork due to the neck of the bottle?
(A) 46 N.
(B) 2.3 x 10^2 N.
(C) 2.7 x 10^2 N.
(D) 1.1 x 10^9 N.
Correct answer: (B)
A shortcut for the differential pressure force is to calculate:
F_diff = delta(P)*A = (5.0 atm)*(101.3 x 10^3 Pa/atm)*pi*(0.012 m)^2,
and setting this equal to the frictional force of the bottleneck required to keep the cord in place. A more thorough approach is calculate the forces due to the pressure both outside of and inside of the bottle:
F_outside = P_outside*A = (1.0 atm)*(101.3 x 10^3 Pa/atm)*pi*(0.012 m)^2,
F_inside = P_inside*A = (6.0 atm)*(101.3 x 10^3 Pa/atm)*pi*(0.012 m)^2,
And thus from Newton's first law:
F_outside + F_friction = F_inside.
Choice (A) is F_outside only. Choice (C) is F_inside only. Choice (D) is delta(P) divided by area.
Student responses
Sections 0906, 0907
(A) : 5 students
(B) : 17 students
(C) : 15 students
(D) : 2 students
Cuesta College, San Luis Obispo, CA
Cf. Giambattista/Richardson/Richardson, Physics, 1/e, Problem 9.78
[3.0 points.] The pressure inside a bottle of champagne is 5.0 atm higher than the air pressure outside. The neck of the bottle has an inner radius of 1.2 cm. What is the frictional force on the cork due to the neck of the bottle?
(A) 46 N.
(B) 2.3 x 10^2 N.
(C) 2.7 x 10^2 N.
(D) 1.1 x 10^9 N.
Correct answer: (B)
A shortcut for the differential pressure force is to calculate:
F_diff = delta(P)*A = (5.0 atm)*(101.3 x 10^3 Pa/atm)*pi*(0.012 m)^2,
and setting this equal to the frictional force of the bottleneck required to keep the cord in place. A more thorough approach is calculate the forces due to the pressure both outside of and inside of the bottle:
F_outside = P_outside*A = (1.0 atm)*(101.3 x 10^3 Pa/atm)*pi*(0.012 m)^2,
F_inside = P_inside*A = (6.0 atm)*(101.3 x 10^3 Pa/atm)*pi*(0.012 m)^2,
And thus from Newton's first law:
F_outside + F_friction = F_inside.
Choice (A) is F_outside only. Choice (C) is F_inside only. Choice (D) is delta(P) divided by area.
Student responses
Sections 0906, 0907
(A) : 5 students
(B) : 17 students
(C) : 15 students
(D) : 2 students
20071113
Physics quiz question: ice skater angular momentum conservation
Physics 5A Quiz 5, Fall Semester 2007
Cuesta College, San Luis Obispo, CA
Cf. Giambattista/Richardson/Richardson, Physics, 1/e, Problem 8.78
[3.0 points.] A figure skater is spinning at a rate of 2.00 rev/s with his arms pulled into his chest. He then extends his arms outwards, increasing his rotational inertia to 150% of its original value. What is the magnitude of his new rate of rotation?
(A) 0.67 rev/s.
(B) 0.89 rev/s.
(C) 1.3 rev/s.
(D) 1.5 rev/s.
Correct answer: (C)
Assume that there are no external torques, or that this process takes a sufficiently short time such that angular momentum is conserved. Then:
L_i = L_f,
I_i*w_i = I_f*w_f,
where 1.5*I_i = I_f. Thus w_f = (I_i/I_f)*w_i = (1/1.5)*w_i.
Student responses
Sections 0906, 0907
(A) : 3 students
(B) : 6 students
(C) : 23 students
(D) : 7 students
Cuesta College, San Luis Obispo, CA
Cf. Giambattista/Richardson/Richardson, Physics, 1/e, Problem 8.78
[3.0 points.] A figure skater is spinning at a rate of 2.00 rev/s with his arms pulled into his chest. He then extends his arms outwards, increasing his rotational inertia to 150% of its original value. What is the magnitude of his new rate of rotation?
(A) 0.67 rev/s.
(B) 0.89 rev/s.
(C) 1.3 rev/s.
(D) 1.5 rev/s.
Correct answer: (C)
Assume that there are no external torques, or that this process takes a sufficiently short time such that angular momentum is conserved. Then:
L_i = L_f,
I_i*w_i = I_f*w_f,
where 1.5*I_i = I_f. Thus w_f = (I_i/I_f)*w_i = (1/1.5)*w_i.
Student responses
Sections 0906, 0907
(A) : 3 students
(B) : 6 students
(C) : 23 students
(D) : 7 students
20071112
Astronomy quiz question: stellar distances
Astronomy 10 Quiz 8, Fall Semester 2007
Cuesta College, San Luis Obispo, CA
Astronomy 10 learning goal Q8.2
Refer to the table below in answering the following question.
[Version 1]
[3.0 points.] Which one of the following choices best corresponds to the star that is the farthest from the Earth?
(A) Sirius.
(B) Canopus.
(C) Capella.
(D) Rigel.
(E) Fomalhaut.
Correct answer: (D)
A star 10 parsecs away will have the same value for apparent and absolute magnitude. A star that is farther than 10 parsecs away will "brighten when brought closer" to the standard 10 parsec distance, and thus its absolute magnitude should be brighter than its apparent magnitude. What is notable for this version of this question is that many students selected Fomalhaut, which most nearly at the standard 10 parsec distance (actually slightly farther than 10 parsecs away).
Student responses
Section 0135
(A) : 2 students
(B) : 3 students
(C) : 1 student
(D) : 9 students
(E) : 14 students
[Version 2]
[3.0 points.] Which one of the following choices best corresponds to the star that is the nearest to the Earth?
(A) Sirius.
(B) Canopus.
(C) Capella.
(D) Rigel.
(E) Fomalhaut.
Correct answer: (A)
A star 10 parsecs away will have the same value for apparent and absolute magnitude. A star that is closer than 10 parsecs away will "dim when pushed away" to the standard 10 parsec distance, and thus its absolute magnitude should be dimmer than its apparent magnitude.
Student responses
Section 1073
(A) : 23 students
(B) : 3 students
(C) : 5 students
(D) : 7 students
(E) : 4 students
Cuesta College, San Luis Obispo, CA
Astronomy 10 learning goal Q8.2
Refer to the table below in answering the following question.
| m apparent magnitude | M absolute magnitude | |
| Sirius | –1.47 | +1.4 |
| Canopus | –0.72 | -3.1 |
| Capella | +0.05 | -0.7 |
| Rigel | +0.14 | -6.8 |
| Fomalhaut | +1.15 | +1.9 |
[Version 1]
[3.0 points.] Which one of the following choices best corresponds to the star that is the farthest from the Earth?
(A) Sirius.
(B) Canopus.
(C) Capella.
(D) Rigel.
(E) Fomalhaut.
Correct answer: (D)
A star 10 parsecs away will have the same value for apparent and absolute magnitude. A star that is farther than 10 parsecs away will "brighten when brought closer" to the standard 10 parsec distance, and thus its absolute magnitude should be brighter than its apparent magnitude. What is notable for this version of this question is that many students selected Fomalhaut, which most nearly at the standard 10 parsec distance (actually slightly farther than 10 parsecs away).
Student responses
Section 0135
(A) : 2 students
(B) : 3 students
(C) : 1 student
(D) : 9 students
(E) : 14 students
[Version 2]
[3.0 points.] Which one of the following choices best corresponds to the star that is the nearest to the Earth?
(A) Sirius.
(B) Canopus.
(C) Capella.
(D) Rigel.
(E) Fomalhaut.
Correct answer: (A)
A star 10 parsecs away will have the same value for apparent and absolute magnitude. A star that is closer than 10 parsecs away will "dim when pushed away" to the standard 10 parsec distance, and thus its absolute magnitude should be dimmer than its apparent magnitude.
Student responses
Section 1073
(A) : 23 students
(B) : 3 students
(C) : 5 students
(D) : 7 students
(E) : 4 students
20071109
Astronomy quiz question: applying the Stefan-Boltzmann law
Astronomy 10 Quiz 8, Fall Semester 2007
Cuesta College, San Luis Obispo, CA
Astronomy 10 learning goal Q8.5
[Version 1]
[3.0 points.] Consider two stars with the same luminosity. Which one of the following choices best describes the star that is larger in size?
(A) The star with the higher surface temperature.
(B) The star with the lower surface temperature.
(C) The star with the brighter apparent magnitude.
(D) The star with the dimmer apparent magnitude.
(E) (None of the above choices (A)-(D), as these two stars must have the same size.)
Correct answer: (B)
The Stefan-Boltzmann law states that luminosity is proportional to size and (temperature)^4. For two stars with the same luminosity, the larger size star must have a lower surface temperature.
Student responses
Section 0135
(A) : 7 students
(B) : 17 students
(C) : 3 students
(D) : 2 students
(E) : 0 students
[Version 2]
[3.0 points.] Consider two stars with the same size. Which one of the following choices best describes the star that has the higher surface temperature?
(A) The star with the brighter apparent magnitude.
(B) The star with the dimmer apparent magnitude.
(C) The star with the brighter luminosity.
(D) The star with the dimmer luminosity.
(E) (None of the above choices (A)-(D), as these two stars must have the same surface temperature.)
Correct answer: (C)
The Stefan-Boltzmann law states that luminosity is proportional to size and (temperature)^4. For two stars of the same size, the brighter luminosity star must have the higher surface temperature.
Student responses
Section 1073
(A) : 6 students
(B) : 1 student
(C) : 31 students
(D) : 3 students
(E) : 1 student
Cuesta College, San Luis Obispo, CA
Astronomy 10 learning goal Q8.5
[Version 1]
[3.0 points.] Consider two stars with the same luminosity. Which one of the following choices best describes the star that is larger in size?
(A) The star with the higher surface temperature.
(B) The star with the lower surface temperature.
(C) The star with the brighter apparent magnitude.
(D) The star with the dimmer apparent magnitude.
(E) (None of the above choices (A)-(D), as these two stars must have the same size.)
Correct answer: (B)
The Stefan-Boltzmann law states that luminosity is proportional to size and (temperature)^4. For two stars with the same luminosity, the larger size star must have a lower surface temperature.
Student responses
Section 0135
(A) : 7 students
(B) : 17 students
(C) : 3 students
(D) : 2 students
(E) : 0 students
[Version 2]
[3.0 points.] Consider two stars with the same size. Which one of the following choices best describes the star that has the higher surface temperature?
(A) The star with the brighter apparent magnitude.
(B) The star with the dimmer apparent magnitude.
(C) The star with the brighter luminosity.
(D) The star with the dimmer luminosity.
(E) (None of the above choices (A)-(D), as these two stars must have the same surface temperature.)
Correct answer: (C)
The Stefan-Boltzmann law states that luminosity is proportional to size and (temperature)^4. For two stars of the same size, the brighter luminosity star must have the higher surface temperature.
Student responses
Section 1073
(A) : 6 students
(B) : 1 student
(C) : 31 students
(D) : 3 students
(E) : 1 student
20071108
Physics clicker question: buoyancy comparison
Physics 5A, Fall Semester 2007
Cuesta College, San Luis Obispo, CA
Cf. Giambattista/Richardson/Richardson, Physics, 1/e, Conceptual Question 9.6 (extended)
Students were asked the following clicker question (Classroom Performance System, einstruction.com) at the beginning of their learning cycle:
Three objects (same volumes, but different densities) float (or do not float) in a tank of water.
[0.6 participation points.] Which object has the greatest weight?
(A) Object X.
(B) Object Y.
(C) Object Z.
(D) (More than one of the objects listed above has the greatest weight.)
(E) (I'm lost, and don't know how to answer this.)
Sections 0906, 0907
(A) : 1 student
(B) : 0 students
(C) : 29 students
(D) : 2 students
(E) : 0 students
Correct answer: (C)
Most students appealed to their intuition to determine that Object Z was the heaviest. Weight force vectors are now drawn on the diagram, and the next question is asked.
[0.6 participation points.] Which object has the greatest bouyant force exerted on it?
(A) Object X.
(B) Object Y.
(C) Object Z.
(D) (More than one of the objects listed above has the greatest bouyant force exerted on it.)
(E) (I'm lost, and don't know how to answer this.)
Sections 0906, 0907
(A) : 22 students
(B) : 3 students
(C) : 2 students
(D) : 7 students
(E) : 0 students
Correct answer: (D)
The magnitude of the bouyant force is the weight of the volume of water displaced. Object X displaces only a portion of its volume, and thus has the least amount of bouyant force exerted on it. Both Objects Y and Z are completely submerged, and thus have the same amount of bouyant force exerted on it. (However, Object Z also has a normal force exerted on it, from the bottom of the tank.)
Cuesta College, San Luis Obispo, CA
Cf. Giambattista/Richardson/Richardson, Physics, 1/e, Conceptual Question 9.6 (extended)
Students were asked the following clicker question (Classroom Performance System, einstruction.com) at the beginning of their learning cycle:
Three objects (same volumes, but different densities) float (or do not float) in a tank of water.
[0.6 participation points.] Which object has the greatest weight?(A) Object X.
(B) Object Y.
(C) Object Z.
(D) (More than one of the objects listed above has the greatest weight.)
(E) (I'm lost, and don't know how to answer this.)
Sections 0906, 0907
(A) : 1 student
(B) : 0 students
(C) : 29 students
(D) : 2 students
(E) : 0 students
Correct answer: (C)
Most students appealed to their intuition to determine that Object Z was the heaviest. Weight force vectors are now drawn on the diagram, and the next question is asked.
[0.6 participation points.] Which object has the greatest bouyant force exerted on it?(A) Object X.
(B) Object Y.
(C) Object Z.
(D) (More than one of the objects listed above has the greatest bouyant force exerted on it.)
(E) (I'm lost, and don't know how to answer this.)
Sections 0906, 0907
(A) : 22 students
(B) : 3 students
(C) : 2 students
(D) : 7 students
(E) : 0 students
Correct answer: (D)
The magnitude of the bouyant force is the weight of the volume of water displaced. Object X displaces only a portion of its volume, and thus has the least amount of bouyant force exerted on it. Both Objects Y and Z are completely submerged, and thus have the same amount of bouyant force exerted on it. (However, Object Z also has a normal force exerted on it, from the bottom of the tank.)
20071107
Physics clicker question: empty can derby
Physics 5A, Fall Semester 2007
Cuesta College, San Luis Obispo, CA
Cf. Giambattista/Richardson/Richardson, Physics, 1/e, Conceptual Example 8.12
"All Disks Roll the Same," 1Q1035.mov
University of Minnesota, School of Physics & Astronomy
http://groups.physics.umn.edu/demo/mechanics/1Q1035.html
Students were asked the following clicker question (Classroom Performance System, einstruction.com) near the end of their learning cycle:
A V-8 can and a Diet Pepsi can (both empty) are placed at the top of an inclined plane, and are released such that they begin to roll down at the same time. (This experiment is set-up, but not yet demonstrated yet for the students until after answers have been compiled.)
[0.6 participation points.] Which empty can do you think will reach downhill first?
(A) V-8 can.
(B) Soda can.
(C) (They will reach the bottom of the slope at approximately the same time.)
(D) (I'm lost, and don't know how to answer this.)
Sections 0906, 0907
(A) : 11 students
(B) : 21 students
(C) : 5 students
(D) : 0 students
Correct answer: (C)
The energy conservation equation for a rolling ring (which approximates an empty beverage can) is:
0 = (1/2)*m*v_f^2 + (1/2)*I*w_f^2 + M*g*h,
where I = m*R^2, such that:
v_f = sqrt(g*h).
Thus both cans will have the same speed as they reach the bottom of the inclined plane, which can plausibly be reasoned to mean that they reach the bottom at the same time.
Cuesta College, San Luis Obispo, CA
Cf. Giambattista/Richardson/Richardson, Physics, 1/e, Conceptual Example 8.12
"All Disks Roll the Same," 1Q1035.movUniversity of Minnesota, School of Physics & Astronomy
http://groups.physics.umn.edu/demo/mechanics/1Q1035.html
Students were asked the following clicker question (Classroom Performance System, einstruction.com) near the end of their learning cycle:
A V-8 can and a Diet Pepsi can (both empty) are placed at the top of an inclined plane, and are released such that they begin to roll down at the same time. (This experiment is set-up, but not yet demonstrated yet for the students until after answers have been compiled.)
[0.6 participation points.] Which empty can do you think will reach downhill first?
(A) V-8 can.
(B) Soda can.
(C) (They will reach the bottom of the slope at approximately the same time.)
(D) (I'm lost, and don't know how to answer this.)
Sections 0906, 0907
(A) : 11 students
(B) : 21 students
(C) : 5 students
(D) : 0 students
Correct answer: (C)
The energy conservation equation for a rolling ring (which approximates an empty beverage can) is:0 = (1/2)*m*v_f^2 + (1/2)*I*w_f^2 + M*g*h,
where I = m*R^2, such that:
v_f = sqrt(g*h).
Thus both cans will have the same speed as they reach the bottom of the inclined plane, which can plausibly be reasoned to mean that they reach the bottom at the same time.
20071106
Physics clicker question: falling stick derby
Physics 5A, Fall Semester 2007
Cuesta College, San Luis Obispo, CA
Cf. Giambattista/Richardson/Richardson, Physics, 1/e, Comprehensive Problems 8.97, 8.98
"Falling Meter Sticks--Scaling," 1Q2060.mov
University of Minnesota, School of Physics & Astronomy
http://groups.physics.umn.edu/demo/mechanics/1Q2060.html
Students were asked the following clicker question (Classroom Performance System, einstruction.com) near the end of their learning cycle:
A 1-m stick and a 2-m stick are held vertically upright, and are released such that they begin to fall over at the same time. (This experiment is set-up, but not yet demonstrated yet for the students until after answers have been compiled.)
[0.6 participation points.] Which stick do you think will fall and hit the ground first?
(A) 1 m stick.
(B) 2 m stick.
(C) (They will hit the ground at approximately the same time.)
(D) (I'm lost, and don't know how to answer this.)
Sections 0906, 0907
(A) : 10 students
(B) : 7 students
(C) : 17 students
(D) : 1 student
Correct answer: (A)
The energy conservation equation for a falling stick is:
0 = (1/2)*I*w_f^2 + M*g*(L/2),
where I = (1/3)*M*L^2, such that:
w_f = sqrt(3*g/L).
Thus the shorter stick will have the faster speed as it hits the ground, which can plausibly be reasoned to be the stick that will reach the ground first, before the longer stick does.
Cuesta College, San Luis Obispo, CA
Cf. Giambattista/Richardson/Richardson, Physics, 1/e, Comprehensive Problems 8.97, 8.98
"Falling Meter Sticks--Scaling," 1Q2060.movUniversity of Minnesota, School of Physics & Astronomy
http://groups.physics.umn.edu/demo/mechanics/1Q2060.html
Students were asked the following clicker question (Classroom Performance System, einstruction.com) near the end of their learning cycle:
A 1-m stick and a 2-m stick are held vertically upright, and are released such that they begin to fall over at the same time. (This experiment is set-up, but not yet demonstrated yet for the students until after answers have been compiled.)
[0.6 participation points.] Which stick do you think will fall and hit the ground first?
(A) 1 m stick.
(B) 2 m stick.
(C) (They will hit the ground at approximately the same time.)
(D) (I'm lost, and don't know how to answer this.)
Sections 0906, 0907
(A) : 10 students
(B) : 7 students
(C) : 17 students
(D) : 1 student
Correct answer: (A)
The energy conservation equation for a falling stick is:0 = (1/2)*I*w_f^2 + M*g*(L/2),
where I = (1/3)*M*L^2, such that:
w_f = sqrt(3*g/L).
Thus the shorter stick will have the faster speed as it hits the ground, which can plausibly be reasoned to be the stick that will reach the ground first, before the longer stick does.
20071105
Lolcat: feline collision
"clunk!" by ridemonkeicanhascheezburger.com
March 27, 2007
Physics 8A learning goal Q8.5
Whether or not the cats rebound from each other or not, momentum would still be conserved, provided that the collision was very brief, and/or that external impulses are negligible.
These cats were apparently posed and photographed from above while sleeping, and not actually photographed while in mid-collision.
20071102
Physics quiz question: elastic collision
Physics 5A Quiz 4, Fall Semester 2007
Cuesta College, San Luis Obispo, CA
Cf. Giambattista/Richardson/Richardson, Physics, 1/e, Problem 7.48
[3.0 points.] A 0.250 kg airtrack cart traveling at 1.50 m/s in the +x direction collides elastically with a stationary cart of 0.075 kg. After they collide, the 0.075 kg cart has a final velocity of +2.31 m/s. Consider frictional forces between the carts and the airtrack to be negligible. What is conserved for this collision?
(A) Momentum only.
(B) Kinetic energy only.
(C) Both momentum and kinetic energy.
(D) (Neither momentum nor kinetic energy are conserved for this collision.)
Correct answer: (C)
Since there are no external horizontal impulses, momentum is conserved. By definition, kinetic energy is conserved for an elastic collision.
Student responses
Sections 0906, 0907
(A) : 4 students
(B) : 6 students
(C) : 28 students
(D) : 2 student
Cuesta College, San Luis Obispo, CA
Cf. Giambattista/Richardson/Richardson, Physics, 1/e, Problem 7.48
[3.0 points.] A 0.250 kg airtrack cart traveling at 1.50 m/s in the +x direction collides elastically with a stationary cart of 0.075 kg. After they collide, the 0.075 kg cart has a final velocity of +2.31 m/s. Consider frictional forces between the carts and the airtrack to be negligible. What is conserved for this collision?
(A) Momentum only.
(B) Kinetic energy only.
(C) Both momentum and kinetic energy.
(D) (Neither momentum nor kinetic energy are conserved for this collision.)
Correct answer: (C)
Since there are no external horizontal impulses, momentum is conserved. By definition, kinetic energy is conserved for an elastic collision.
Student responses
Sections 0906, 0907
(A) : 4 students
(B) : 6 students
(C) : 28 students
(D) : 2 student
20071101
Physics quiz question: bullet into block
Physics 5A Quiz 4, Fall Semester 2007
Cuesta College, San Luis Obispo, CA
Cf. Giambattista/Richardson/Richardson, Physics, 1/e, Problem 7.43
[3.0 points.] A block of wood of 1.20 kg is initially at rest. A bullet of mass 0.030 kg traveling at 180 m/s in the +x direction strikes the block and becomes embedded in it. Consider the frictional force between the block and the ground to be negligible. What is conserved for this collision?
(A) Momentum only.
(B) Kinetic energy only.
(C) Both momentum and kinetic energy.
(D) (Neither momentum nor kinetic energy are conserved for this collision.)
Correct answer: (A)
Since there are no external horizontal impulses, momentum is conserved. However, since there is deformation with no rebound and separation in this perfectly inelastic collision, kinetic energy is not conserved.
Student responses
Sections 0906, 0907
(A) : 26 students
(B) : 6 students
(C) : 7 students
(D) : 1 student
Cuesta College, San Luis Obispo, CA
Cf. Giambattista/Richardson/Richardson, Physics, 1/e, Problem 7.43
[3.0 points.] A block of wood of 1.20 kg is initially at rest. A bullet of mass 0.030 kg traveling at 180 m/s in the +x direction strikes the block and becomes embedded in it. Consider the frictional force between the block and the ground to be negligible. What is conserved for this collision?
(A) Momentum only.
(B) Kinetic energy only.
(C) Both momentum and kinetic energy.
(D) (Neither momentum nor kinetic energy are conserved for this collision.)
Correct answer: (A)
Since there are no external horizontal impulses, momentum is conserved. However, since there is deformation with no rebound and separation in this perfectly inelastic collision, kinetic energy is not conserved.
Student responses
Sections 0906, 0907
(A) : 26 students
(B) : 6 students
(C) : 7 students
(D) : 1 student
Subscribe to:
Posts (Atom)