Cuesta College, San Luis Obispo, CA
Students have a bi-weekly online reading assignment (hosted by SurveyMonkey.com), where they answer questions based on reading their textbook, material covered in previous lectures, opinion questions, and/or asking (anonymous) questions or making (anonymous) comments. Full credit is given for completing the online reading assignment before next week's lecture, regardless if whether their answers are correct/incorrect. Selected results/questions/comments are addressed by the instructor at the start of the following lecture.
The following questions were asked on reading textbook chapters and previewing a presentation on ideal fluid flow.

Selected/edited responses are given below.
Describe what you understand from the assigned textbook reading or presentation preview. Your description (2-3 sentences) should specifically demonstrate your level of understanding.
"An ideal liquid is one that is in-compressible, non-viscous, and should undergo a laminar flow. The conservation laws regarding liquids allow us to determine several factors regarding liquids including volume and energy."
"The differences between compressible and non-compressible fluids which is kind of straight forward. Also the difference between non-viscous and viscous and between laminar and turbulent."
"An ideal fluid is incompressible, laminar, and non-viscous. Since it is incompressible, volume flow rate is conserved. Even when a pipe changes radius, the incompressibility of an ideal fluid means the same volume flowing in one end equals the same volume coming out the other end in the same time interval."
"I feel like I have a good grasp on the continuity equation. If the area of the 'in' is smaller than the area of the 'out,' then the speed will decrease on the way out. If the area for the 'in' is bigger than the 'out,' then the speed will increase on the way out."
"The volume flow rate of a fluid is defined to be the volume of fluid that is passing through a given cross sectional area per unit time. Because liquids are incompressible, any portion of liquid flowing through a pipe could change shape, but it must maintain the same volume. This is true even if the pipe changes diameter. In the diagram below [for the horizontal narrowing pipe] of liquid on the left changes shape as it enters a narrow section of pipe, but it maintains the same volume since liquids are incompressible."
"Sorry P-dog, but I'm still preparing for my art history midterm."
Describe what you found confusing from the assigned textbook reading or presentation preview. Your description (2-3 sentences) should specifically identify the concept(s) that you do not understand.
"I am kind of confused about Bernoulli's equation."
"I need help understanding Bernoulli's equation."
"I cannot seem to understand energy density conservation."
"I was confused why the equations have to balance out all the time."
"I need a better understanding of what each of the symbols represent in the equations. as well how to properly go about solving them."
"I found Bernoulli's equation a bit confusing. Mostly how gravitational potential energy density is affected by the cross-sectional area."
"I found the energy density conservation equations kind of confusing and would like to see examples of them worked out in lecture."
"Bernoulli's equation--I am not sure if the equation will always be balanced or if there are some cases where the right-hand side will not equal to 0."
"I think I understand why area and fluid speed increase, decrease, or are constant for given situations but I'm a little unsure when it comes to determining the values of each term in Bernoulli's equation. "
"I understand the difference between viscous and non-viscous liquids. I found everything else about this reading confusing."
"It all makes sense."
"I think I'm going to be okay right now."
What is the SI (Système International) unit for volume flow rate?
"m3/s."
"Cubic meters per second."
"kg/s?"
"m/s2?"
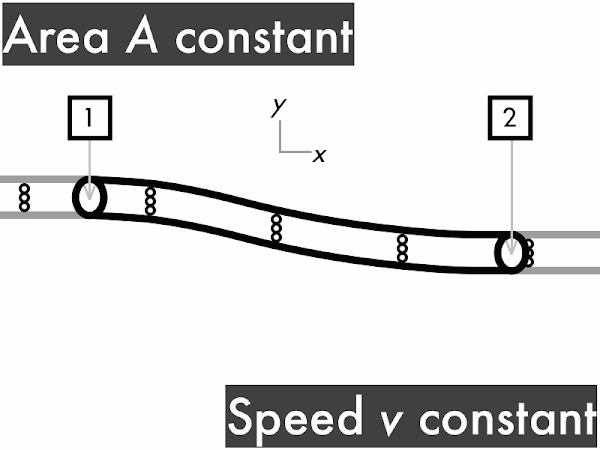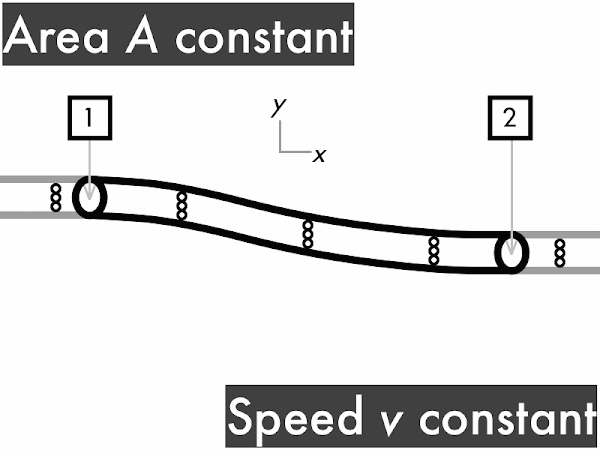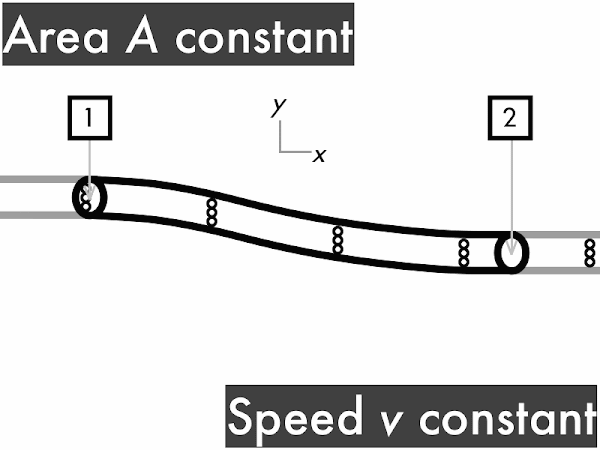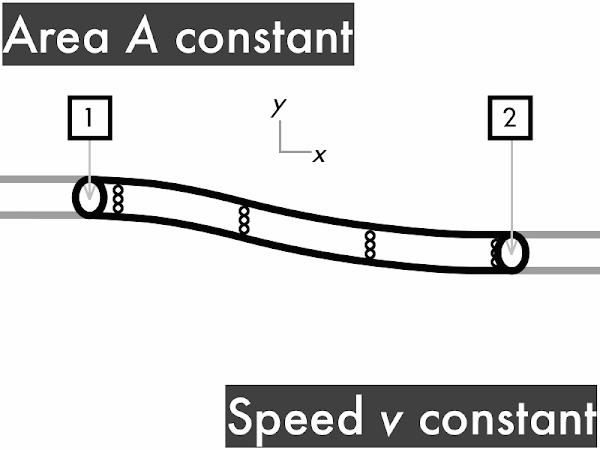
decreases. [0] remains constant. *********************************************** [47] increases. ** [2] (Unsure/lost/guessing/help!) * [1]

decreases. ******************** [20] remains constant. *********************** [23] increases. ****** [6] (Unsure/lost/guessing/help!) * [1]
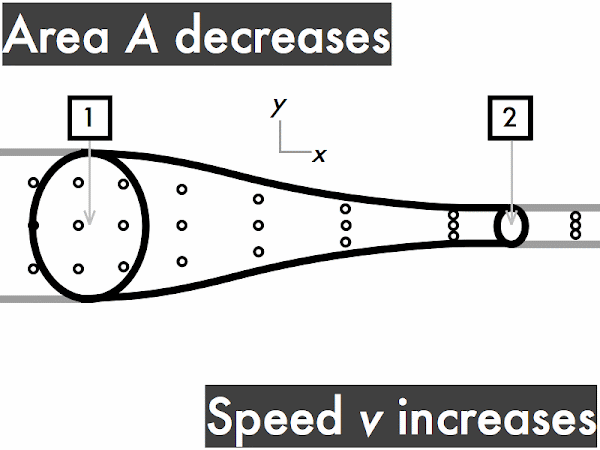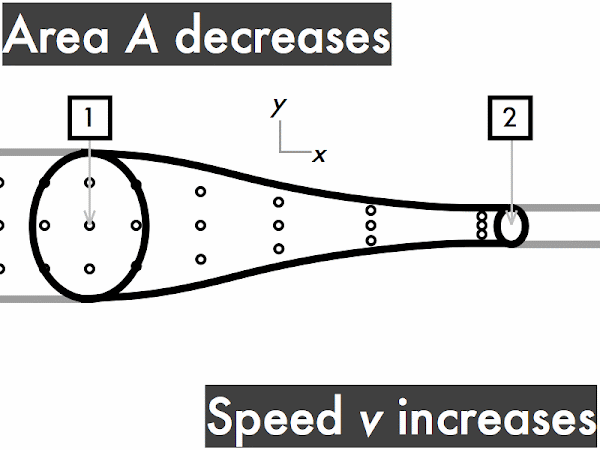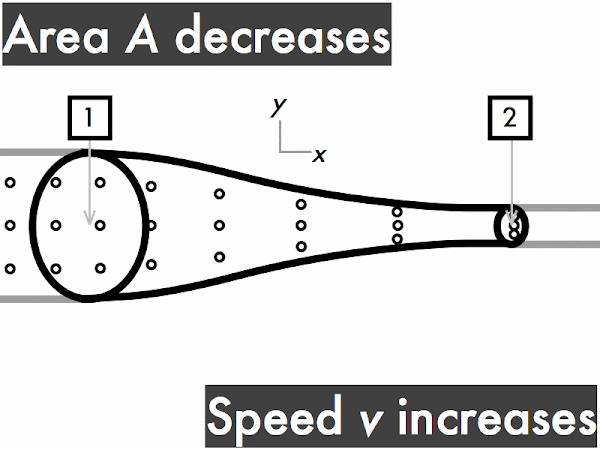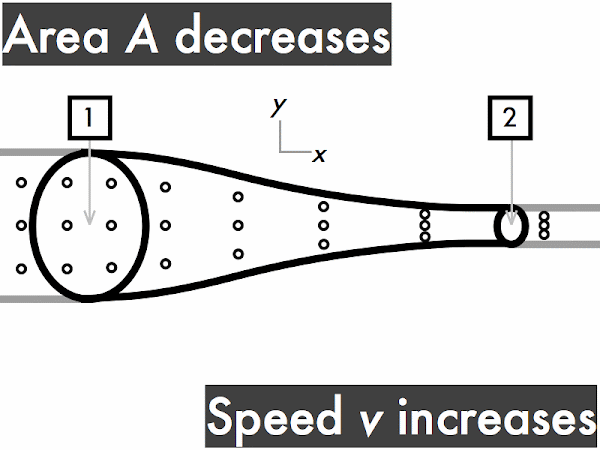
decreases. ******* [7] remains constant. ********************* [21] increases. ********************* [21] (Unsure/lost/guessing/help!) * [1]

(Only correct responses shown.)
(1/2)·ρ·∆(v2): decreases [58%]
ρ·g·∆y: no change [64%]
∆P: increases [52%]

(Only correct responses shown.)
(1/2)·ρ·∆(v2): increases [54%]
ρ·g·∆y: no change [56%]
∆P: decreases [54%]

(Only correct responses shown.)
(1/2)·ρ·∆(v2): no change [72%]
ρ·g·∆y: decreases [26%]
∆P: increases [18%]
Ask the instructor an anonymous question, or make a comment. Selected questions/comments may be discussed in class.
"When you say water is incompressible to some extent, is that referring to ice?" (And liquid water, as well. This is why hydraulics work, as well as intravenous drips and hypodermic injections--push in here, stuff on the other side pushes out.)
"Is the flow rate with an ideal fluid always constant with the in and out?" (Yes, as ideally the fluid would be incompressible.)
"A little lost on the potential changes in kinetic, gravitational, pressure changes for the this last pipe with what appears to be no change in cross-sectional area, but a decrease in gravitational energy density." (That sounds pretty good, though.)
"I am a little confused on the descending pipe question and whether the pressure increases or decreases." (The pressure will increase, as the gravitational potential energy density decreases.)
"This is very difficult."
"I would like to go over these laws more in class."
"I don't understand the variables, but I believe that I will understand the concepts once we clarify each variable."
"Doing good so far."
"How do you have time to read 60+ comments?" (If I ask 60+ students to make time to answer questions and/or make comments on the reading assignments, then I have to make time to read them all. #becarefulofwhatyouwishfor)
No comments:
Post a Comment